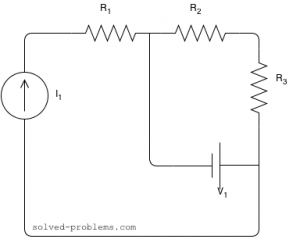
Find the voltage across the current source and the current passing through the voltage source.
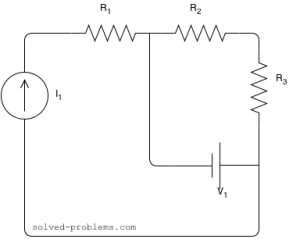
Problem 1213
Assume that I_1=3A, R_1=2 \Omega, R_2=3 \Omega, R_3=2 \Omega,I_1=3A, V_1=15 V,

Solution
R_1 is in series with the current source; therefore, the same current passing through it as the current source:

I_{R_1}=I_1=3A
and the voltage across R_1 can be found by Ohm’s law:
V_{R_1}=R_1 \times I_{R_1} =2 \times 3 = 6V
To find the voltage across the current source, KVL can be applied around the left hand side loop:
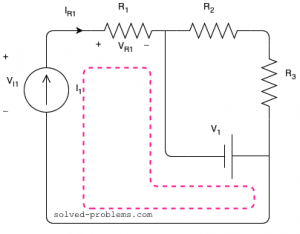
Problem 1213 -5
The direction does not matter and would not change the result.
-V_{I_1}+V_{R_1}-V_1=0A
V_{I_1}=V_{R_1}-V_1=6-15=-9 V.

R_2 and R_3 are also in series and their equivalent is R_{23}=R_2+R_3=3 \Omega + 2 \Omega=5 \Omega
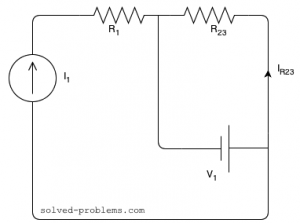
Problem 1213 - 3

R_{23} is parallel with the voltage source. This means that its voltage is equal to the voltage of the voltage source.
V_{R_{23}}=V_1=15 V
Now, using the Ohm’s law, the current passing through R_{23} can be calculated:
I_{R_{23}}=\frac{V_{R_{23}}}{R_{23}}=\frac{15}{5}=3 A
To find the current of the voltage source, we can apply KCL at one of the nodes:
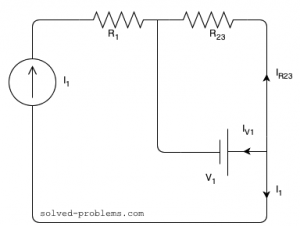
Problem 1213 - 4

I_{R_{23}}+I_1+I_{V_1}=0

I_{V_1}=-I_{R_{23}}-I_1=-3-3=-6 A
Now, you tell me below what the power of each sources are?

Published by Yaz

Hi! Yaz is here. I am passionate about learning and teaching. I try to explain every detail simultaneously with examples to ensure that students will remember them later too.

Join the Conversation

5 Comments

  1. Reply
    1. Reply
  2. Reply
  3. Reply
  4. Reply

Leave a comment

Your email address will not be published. Required fields are marked *

This site uses Akismet to reduce spam. Learn how your comment data is processed.